CBB: How Often Does the Nation’s Best Team Win the National Championship?
Posted by Neil Paine on March 31, 2010
Note: This post was originally published at College Basketball Reference, S-R's brand-new College Hoops site, so when you're done reading, go over and check it out!
In a variation on a running theme (one that's especially pertinent given the early departures of Syracuse, Kansas, & Kentucky from this year's tourney), I wanted to know how often the "best" (i.e., most talented, most dominant over the entire season, etc.) team wins the NCAA Tournament. We know that the NFL's best team wins the Super Bowl about 24% of the time, that the best team in baseball wins the World Series about 29% of the team (or at least, they did back in the 1980s when Bill James studied the issue), and that the NBA's best team wins the Finals almost half of the time... So what's your guess for college basketball?
On the one hand, the NCAA tourney seems to have more in common with football, since it's a string of single-elimination games (made even more random by the games being played at neutral sites); however, on the other hand it's still basketball, and the structure of the game itself is set up such that the better team wins more often than in other sports (in no small part due to the massive number of possessions per game -- unlike football -- as well as the ability to have your best player carry a disproportionate share of the team's offensive chances -- unlike baseball).
But rather than just make a guess, I once again set up a Monte Carlo simulator and ran a series of "test seasons". To do this, I used the 2009-10 D-I basketball schedule, excluding postseason & conference tournament games, as well as games involving non-D-I schools. For each school, I assigned them a random "strength rating" from a normal distribution based on the average SRS scores of their conference members since 1980:
| Conf | avgSRS | stdvSRS |
|---|---|---|
| A10 | 2.96 | 7.01 |
| ACC | 12.18 | 7.08 |
| AMEA | -8.87 | 5.70 |
| ASUN | -7.79 | 7.02 |
| BG10 | 11.54 | 7.09 |
| BG12 | 9.23 | 7.37 |
| BIGE | 10.06 | 6.52 |
| BSKY | -4.49 | 6.63 |
| BIGS | -11.15 | 5.62 |
| BIGW | -1.93 | 6.35 |
| CAA | -3.43 | 6.81 |
| CUSA | 3.01 | 7.43 |
| GWC | -9.58 | 7.28 |
| HRZN | -2.21 | 6.44 |
| IND | -19.09 | 9.72 |
| IVY | -7.93 | 6.62 |
| MAAC | -5.15 | 6.03 |
| MAC | -0.93 | 6.24 |
| MEAC | -14.77 | 6.41 |
| MVC | 2.53 | 5.90 |
| MOUW | 5.08 | 6.93 |
| NEAS | -10.69 | 5.65 |
| OVC | -6.94 | 5.91 |
| PC10 | 9.22 | 6.87 |
| PATR | -8.36 | 6.52 |
| SUNB | -2.83 | 6.86 |
| SOUC | -6.14 | 6.26 |
| SEC | 10.76 | 5.91 |
| STHL | -8.13 | 6.34 |
| SUML | -5.73 | 5.57 |
| SWAC | -13.93 | 7.51 |
| WAC | 1.48 | 6.01 |
| WCC | 0.26 | 6.70 |
So for instance, you might end up with an ACC that looks like this:
| Team | Conf | Strength |
|---|---|---|
| North Carolina St | ACC | 21.61 |
| Virginia Tech | ACC | 21.36 |
| Wake Forest | ACC | 19.58 |
| Duke | ACC | 16.01 |
| Maryland | ACC | 12.81 |
| Boston College | ACC | 11.65 |
| Florida St | ACC | 7.82 |
| Miami FL | ACC | 7.80 |
| Georgia Tech | ACC | 4.18 |
| Virginia | ACC | 3.39 |
| North Carolina | ACC | 3.21 |
| Clemson | ACC | -1.13 |
...And a Patriot League that looks like this:
| Team | Conf | Strength |
|---|---|---|
| Holy Cross MA | Pat | 0.07 |
| Colgate | Pat | -5.19 |
| Lehigh | Pat | -5.54 |
| Bucknell | Pat | -6.11 |
| Navy | Pat | -7.10 |
| Army | Pat | -7.14 |
| Lafayette | Pat | -9.75 |
| American U | Pat | -12.34 |
Do this for every team in the country, and estimate the chances of one team beating another at home using this equation:
p(homeWin) = 1 / (1 + EXP(-0.7390072 - 0.179593 * SRSdiff))
(Note: For neutral games, I averaged p(homeWin) and p(roadWin) in a given matchup.)
From this, you can generate standings for the 2010 regular season, like these:
| Team | Conf | W | L | W% | confW | confL | confW% |
|---|---|---|---|---|---|---|---|
| California | P10 | 28 | 2 | 0.933 | 16 | 2 | 0.889 |
| Washington St | P10 | 23 | 6 | 0.793 | 12 | 6 | 0.667 |
| Washington | P10 | 23 | 7 | 0.767 | 12 | 6 | 0.667 |
| Oregon St | P10 | 20 | 10 | 0.667 | 11 | 7 | 0.611 |
| Oregon | P10 | 20 | 10 | 0.667 | 9 | 9 | 0.500 |
| Stanford | P10 | 17 | 13 | 0.567 | 8 | 10 | 0.444 |
| UCLA | P10 | 17 | 13 | 0.567 | 8 | 10 | 0.444 |
| Arizona St | P10 | 15 | 16 | 0.484 | 7 | 11 | 0.389 |
| Arizona | P10 | 8 | 22 | 0.267 | 5 | 13 | 0.278 |
| Southern Cal | P10 | 6 | 24 | 0.200 | 2 | 16 | 0.111 |
You can also use these simulated W-L results to calculate RPI, the Ratings Percentage Index that the selection committee uses to inform their picks. The formula is 25% team winning pct., 50% opponents' winning pct., and 25% opponents' opponents' winning pct:
| Team | Conf | W% | Opp% | Opp^2% | RPI |
|---|---|---|---|---|---|
| Arkansas | SEC | 0.968 | 0.542 | 0.538 | 0.648 |
| Kentucky | SEC | 0.871 | 0.554 | 0.544 | 0.631 |
| LSU | SEC | 0.767 | 0.568 | 0.546 | 0.612 |
| Mississippi | SEC | 0.800 | 0.528 | 0.548 | 0.601 |
| South Carolina | SEC | 0.700 | 0.550 | 0.543 | 0.586 |
| Vanderbilt | SEC | 0.690 | 0.547 | 0.544 | 0.582 |
| Florida | SEC | 0.548 | 0.567 | 0.539 | 0.555 |
| Georgia | SEC | 0.517 | 0.575 | 0.545 | 0.553 |
| Alabama | SEC | 0.467 | 0.593 | 0.535 | 0.547 |
| Tennessee | SEC | 0.467 | 0.589 | 0.539 | 0.546 |
| Mississippi St | SEC | 0.419 | 0.559 | 0.530 | 0.517 |
| Auburn | SEC | 0.367 | 0.580 | 0.525 | 0.513 |
Now we have all the components in place to put together the field for a simulated NCAA Tournament. The selections go like this: the 32 conference regular-season champions get automatic bids (ties are broken by RPI); the remaining 32 at-large slots are filled by the 32 best non-conference champs by RPI. Overall seedings are then made, ranking all tourney selections by RPI, and the regions are seeded according to an S-curve.
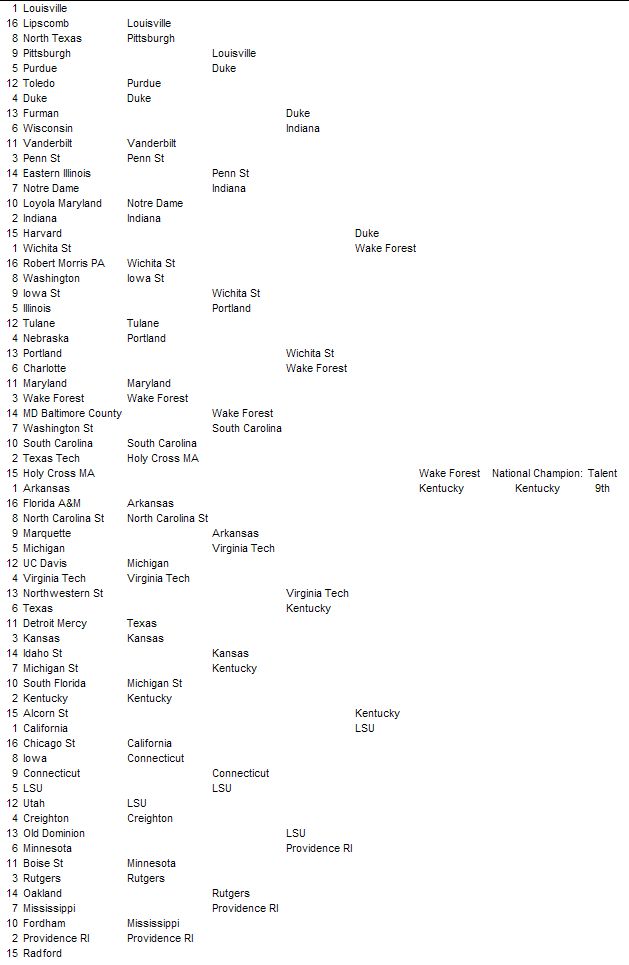
As you can see, I tracked the global "talent ranking" of the eventual champion for each simulated season; in this case, it was Kentucky, who ranked 9th in team talent (in case you're wondering, NC State was #1, but they were toppled by Arkansas in the Round of 32). Repeat this process 1,000 times, and how often does a team of each talent ranking win it all?
| Ranking | Championships |
|---|---|
| 1 | 336 |
| 2 | 165 |
| 3 | 103 |
| 4 | 79 |
| 5 | 62 |
| 6 | 35 |
| 7 | 37 |
| 8 | 27 |
| 9 | 34 |
| 10 | 18 |
| 11 | 15 |
| 12 | 16 |
| 13 | 13 |
| 14 | 9 |
| 15 | 6 |
| 16 | 9 |
| 17 | 6 |
| 18 | 2 |
| 19 | 2 |
| 20 | 4 |
| 21 | 1 |
| 22 | 2 |
| 23 | 5 |
| 24 | 2 |
| 25 | 1 |
| 26 | 1 |
| 27 | 0 |
| 28 | 4 |
| 29 | 0 |
| 30 | 1 |
| 31 | 2 |
| 32 | 0 |
| 33 | 1 |
| 34 | 0 |
| 35 | 1 |
| 36 | 0 |
| 37 | 0 |
| 38 | 1 |
The nation's most talented team wins the National Championship approximately one-third of the time in this simulation, which is slightly higher than we observed for football and baseball, but much lower than the results of a similar simulation of NBA results. Going back to our original hypotheses, it was clear that a single-elimination tournament of 6 rounds creates many opportunities for favored teams to slip up along the way to a title, in much the same way that the NFL playoffs' one-and-done format encourages parity. At the same time, though, the better team wins a basketball game more often than it wins a football or baseball game simply because the random variance of luck is reduced with higher-scoring games and more possessions.
In the end, we saw basically what we should have predicted before the experiment -- by mixing high-variance elements of other playoffs, including a single-elimination format, the NCAA Tournament will never see the best team prevail as often as we see in the NBA Playoffs, but the nature of basketball offsets these elements to a certain degree, resulting in a winning percentage for the best team that falls between those of the NBA and the NFL/MLB.
And a less abstract take-home message? Well, when the 2010 NCAA champs are crowned on Monday night, they'll be the most deserving team, having outlasted 64 others over the past few weeks... But will they be the nation's best college basketball team? The odds have spoken -- and they say probably not.

March 31st, 2010 at 5:19 am
You have Michigan (undergrad) with a better seed than Michigan State (or even in the tournament), and you let Toledo (grad) and Tulane (rest of family) into the tournament. I have no problem with any of this.
March 31st, 2010 at 10:47 am
Fantastic work, Neil. I'm actually shocked that it's that high. I would have guessed 10--15%.
March 31st, 2010 at 5:26 pm
I finally figured out what you meant about simulating records, but along the way discovered that the last time Cal went 28-2 was in 1960, when as the defending champs they lost the NCAA final to Lucas & Havlicek.
The only way you're liable to find Darrall Imhoff without a picture of Wilt dropping 100 on him.
November 15th, 2011 at 7:36 pm
Howdy! Quick question that's entirely off topic. Do you know how to make your site mobile friendly? My site looks weird when viewing from my apple iphone. I'm trying to find a template or plugin that might be able to fix this issue. If you have any recommendations, please share. Thanks!
November 18th, 2011 at 7:41 am
Thanks for sharing excellent informations. Your site is so cool. I'm impressed by the details that youˇ¦ve on this blog. It reveals how nicely you perceive this subject. Bookmarked this web page, will come back for extra articles. You, my friend, ROCK! I found just the info I already searched all over the place and simply could not come across. What a great site.